


Mathematical Models
807 kr
807 kr
På lager
On., 29 jan. - ti., 4 feb.
Sikker betaling
14 dagers åpent kjøp
Selges og leveres av
Adlibris
Produktbeskrivelse
Mathematics is a grand subject in the way it can be applied to various problems in science and engineering. To use mathematics, one needs to understand the physical context. The author uses mathematical techniques along with observations and experiments to give an in-depth look at models for mechanical vibrations, population dynamics, and traffic flow. Equal emphasis is placed on the mathematical formulation of the problem and the interpretation of the results. In the sections on mechanical vibrations and population dynamics, the author emphasizes the nonlinear aspects of ordinary differential equations and develops the concepts of equilibrium solutions and their stability. He introduces phase plane methods for the nonlinear pendulum and for predator-prey and competing species models. Haberman develops the method of characteristics to analyze the nonlinear partial differential equations that describe traffic flow. Fan-shaped characteristics describe the traffic situation that occurs when a traffic light turns green and shock waves describe the effects of a red light or traffic accident. Although it was written over 20 years ago, this book is still relevant.It is intended as an introduction to applied mathematics, but can be used for undergraduate courses in mathematical modeling or nonlinear dynamical systems or to supplement courses in ordinary or partial differential equations.
Artikkel nr.
ea03365e-c969-493c-a27b-9ab513745c85
Mathematical Models
807 kr
807 kr
På lager
On., 29 jan. - ti., 4 feb.
Sikker betaling
14 dagers åpent kjøp
Selges og leveres av
Adlibris
Lignende toppselgere

Generic
Øreputer for Bose QuietComfort - QC35/QC25/QC15/AE2 Hodetelefoner Svart
99 kr
4,5(717)
mandag, 27 jan.

Megabilligt
Galaxy Lamp - Nebula Star -projektoren med fjernkontroll
499 kr
Tidligere laveste pris:
909 kr
3,8(28)
mandag, 27 jan.

CherrysC
Squid Game 2 Gonggi & Case Korean
129 kr
fredag, 31 jan.

INF
Universallader for Garmin klokker
90 kr
4,3(262)
fredag, 24 jan.

Odin
Titan Silikon Til Tredemølle 100ml
149 kr
Tidligere laveste pris:
349 kr
4,3(7)
fredag, 24 jan.

Generic
2-Pak - iPhone Lader Adapter+Kabel 20W USB-C Hurtiglader
199 kr
3,7(503)
mandag, 27 jan.

Maaleo
Dobbeltsidige tusjer/penner - 168 stk. MAALEO 24101
399 kr
2,3(3)
torsdag, 6 feb.

INF
INF Sklisikker beskyttelse / brodder for sko med 18 stålpigger (L)
259 kr
Tidligere laveste pris:
291 kr
4,4(22)
torsdag, 23 jan.

Generic
2-Pak - PS4 Kontroll DoubleShock for Playstation 4 - Trådløs
549 kr
4,1(9)
mandag, 27 jan.
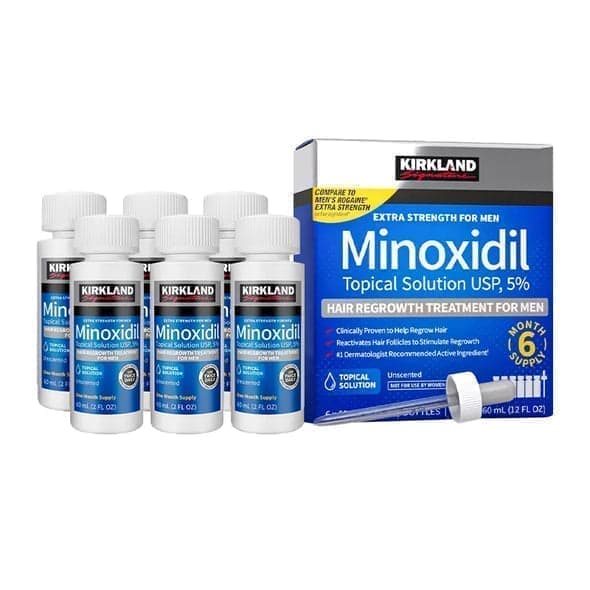
KIRKLAND
6x60ml = 360ml - Kirkland Extra Strength 5% Men Hair Regrowth 60ml Hair Loss
1 290 kr
5,0(5)
fredag, 24 jan.
Anbefalinger til dig

INF
INF Stylus Pen kompatibel med iPad 2018-2023-serien HvitiPad
196 kr
Tidligere laveste pris:
268 kr
4,1(43)
fredag, 24 jan.

PlayStation 5 Slim Digital Edition (PS5)
5 789 kr
4,4(38)
fredag, 24 jan.

Mobil o Teknik
Elektrisk babyneglefil - fra nyfødt til småbarn
199 kr
4,0(5)
tirsdag, 28 jan.

Toppik
Toppik - 27,5g - Dark Brown - Mørkebrun
285 kr
4,4(11)
fredag, 24 jan.
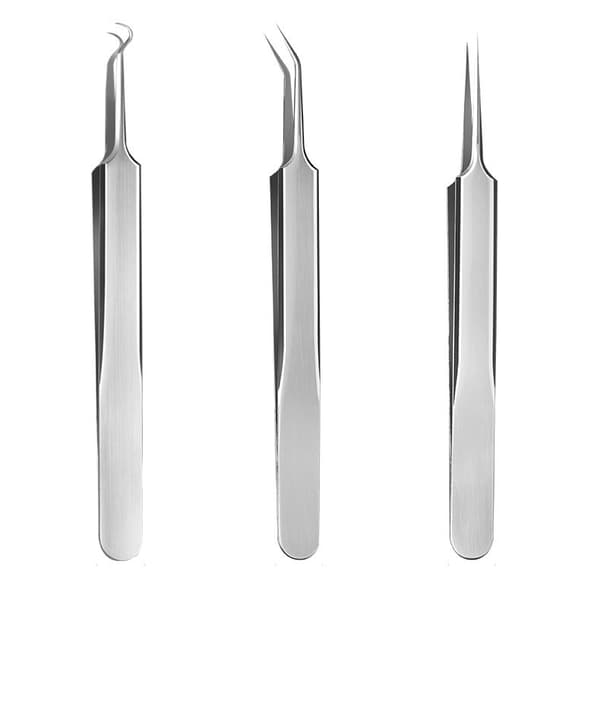
INF
INF Hudormfjerner i rustfritt stål 3 deler Sølv
106 kr
5,0(4)
fredag, 24 jan.

INF
INF Ankelstropp for styrketrening med kabelmaskiner 2 Pack
147 kr
Tidligere laveste pris:
149 kr
4,4(5)
fredag, 24 jan.

Nördic
NÖRDIC 192kHz DAC Digital til Analog Converter SPDIF Toslink til Analog Stereo RCA L/R
350 kr
4,1(14)
fredag, 24 jan.

INF
INF Babymonitor med kamera 2.4G-overføring, 2-veis lyd, infrarødt nattsyn
819 kr
4,0(5)
torsdag, 23 jan.

INF
INF Sett for bartending med dobbel shaker 750ml i rustfritt stål, 10 redskaper
272 kr
Tidligere laveste pris:
397 kr
4,5(57)
torsdag, 23 jan.
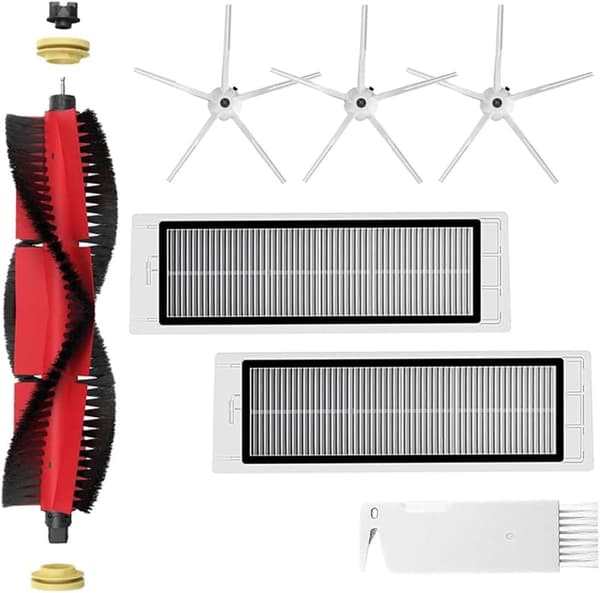
INF
INF Tilbehør til Roborock S5/S6 modeller 7 deler
219 kr
4,2(49)
torsdag, 23 jan.